Place Value from the Ground Up is the second textbook that we have published for the iPad through the iBookstore using Apple’s iBooks Author software. This textbook immerses students in a microworld of blocks and groups of blocks
Coined by Seymour Papert in 1980, microworlds are self-contained worlds that students learn about simply by exploring, navigating, and solving problems within the microworld itself. Just like an athlete learns the rules of soccer while playing soccer, but does not play soccer to learn the rules of soccer, students engage in microworlds to solve puzzles and overcome challenges, not to learn a specific set of content.
This does not mean that a microworld attempts to disguise learning or trick students into learning. The purpose of a microworld is to leverage highly-evolved skills that humans have developed to learn about and make sense of the world around us. We do this naturally. A microworld also puts students in the moment, which means focused on the task at hand instead of worrying about what they are or are not learning.
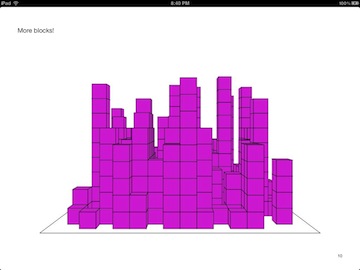
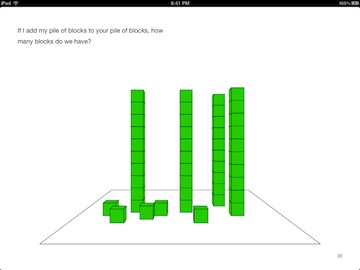
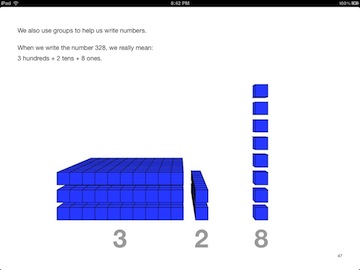
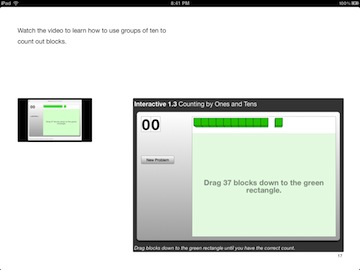
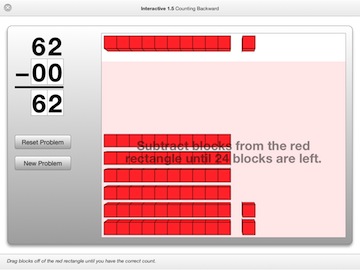
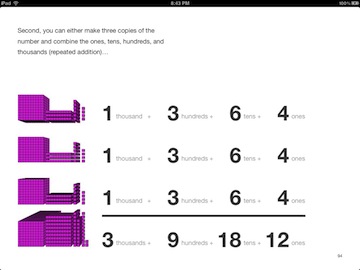
In this textbook, students are immersed in a microworld where they are asked to count blocks. As the blocks they need to count become more numerous, they discover that grouping the blocks can make their lives easier. However, grouping the blocks also increases the organizational overhead and cognitive load of the puzzles. The need to keep track of groups (and groups of groups) leads to an understanding of place value and its applications.
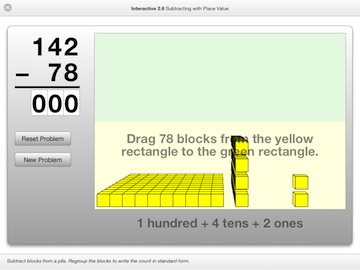
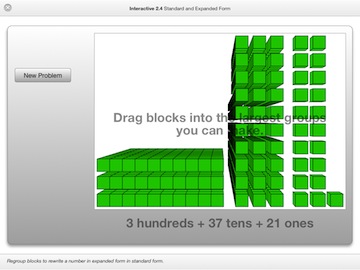
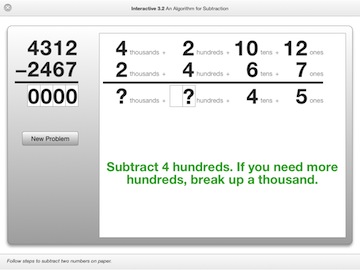
Because students are immersed in a microworld, this textbook does not follow a traditional learning sequence for place value. For example, it does not explicitly introduce the concept of place value until the second chapter (later than usual) and it encourages students to apply place value concepts to subtraction, multiplication, and division in the first chapter (much earlier than usual).
The first chapter of the textbook is highly concrete and is geared toward first graders. At this stage, students are using trial and error and solutions tend to be “ad hoc” rather than systematic. This means that the student does something, studies the result, and then figures out on the spot what to do next. There is little strategy or planning involved, and they may not solve a problem the same way twice. The second chapter is geared toward second graders. Students at this stage are beginning to develop basic theories and strategies for working with groups. Now they know they can solve the problems, and their goal is to solve the problems more efficiently. In the third chapter, geared toward third graders, students begin to generalize. Instead of being immersed in the physical world of blocks, they are immersed in a slightly more abstract world of numbers (the blocks are still only a mental visualization or quick sketch away). And finally, the fourth chapter lays down some bread crumbs that an inquisitive student may find intriguing and worthy of exploration.
The immersive learning in this textbook is meant to supplement direct instruction, not completely replace it. Students still need someone to help them formalize and test their theories. A younger student might use this textbook to begin developing place value concepts before they see them in school. An older student might use this textbook to make the connections that he or she missed the first time around. The microworlds in this textbook can also be used as a vehicle for direct instruction if integrated into a full curriculum.
Right now this textbook is specifically targeting the home/iPad market. However, we recognize that not everyone has access to an iPad and that this curriculum can have a wider impact if implemented in schools. The microworlds in this textbook have been built using javascript + html + css, so if there is sufficient interest, we would be willing to release a web-based version of this textbook, especially for school districts.